Answer:
2.65 inches
Explanation:
The length of the toothpick is 3 inches.
The of the base of the box is 1 inch x 1 inch.
Let the height of the box is h inch, so, the dimension of the box is
1 inch x 1 inch x h inch.
The toothpick must be placed diagonally for the minimum height of the box, as shown in the figure,
So, the length of the longest diagonal of the box
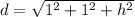
As the length of the toothpick is 3 inches, so d= 3
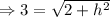
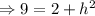

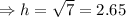 inches.
inches.
Hence, the shortest possible length of the box is 2.65 inches.