Answer:
The speed of the car is 21.91 m/s
Explanation:
Centripetal Acceleration
It's the acceleration experienced by an object in a uniform circular motion.
It always points toward the center of rotation and is perpendicular to the linear velocity v.
The magnitude of the centripetal acceleration is:
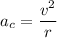
Where r is the radius of rotation.
The race car has a centripetal acceleration of
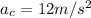 and the radius of rotation is r=40 m.
and the radius of rotation is r=40 m.
We can calculate the speed of the car by solving the above equation for v:
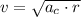
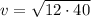

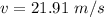
The speed of the car is 21.91 m/s