Question

These are the two I need help with:

4. Find the sample standard deviation, sample variance, and range for your survey responses.
5. Find the z-score for the largest (maximum) value in your data set. Is that value an outlier?


This is the data and answers so far

0 0 2 2 2 2 2 2 3 3 3 3 4 4 5 6 7 7 7 8 21
3. Mean- 4.428571429
Median- 3
Mode- 2
Range- 21

4. Standard Deviation- 4.4336376551476
Sample Variance- 19.657142857143
Range-

5. Z score

Answer 1

Z-score = 3.73

Yes, the maximum value (21) in this data set is a high outlier.

Explanation:

I'll solve for what you haven't done yet, z-score and whether or not the maximum is an outlier.

Z-score tells us how many standard deviations a value is above or below the mean. The formula for z-score is
`\displaystyle z=(x_i-\mu)/(\sigma)`.

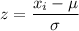Substituting
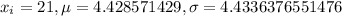`x_i=21, \mu = 4.428571429, \sigma=4.4336376551476`, we get
`z\approx 3.73`.

To determine if a value is an outlier, we use IQR, or Interquartile Range. If a value is lower than
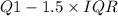`Q1-1.5* IQR` or higher than
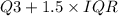`Q3+1.5* IQR`, then we say it is an outlier.

With the value of 21, clearly we are only worried about it being a high outlier. Q1 is the median of the first half of the data and Q3 is the median of the second half. In this case, Q1 is 2 and Q3 is 6.5. IQR is equal to Q3-Q1, or 4.5 in this case.

Therefore, the higher limit for outliers is
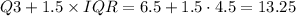`Q3+1.5* IQR=6.5+1.5\cdot 4.5=13.25`. Any values above 13.25 are considered high outliers. Therefore, the maximum value of 21 is a high outlier.