Question

PLEASE HELP ME GUYS OR I WONT PASS
this calculus!!!!​

Answer 1

b.
`\displaystyle (1)/(2)`

General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Algebra I

• Functions
• Function Notation
• Exponential Rule [Rewrite]:
  `\displaystyle b^(-m) = (1)/(b^m)`
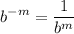
• Exponential Rule [Root Rewrite]:
  `\displaystyle \sqrt[n]{x} = x^{(1)/(n)}`

Calculus

Derivatives

Derivative Notation

Basic Power Rule:

• f(x) = cxⁿ
• f’(x) = c·nxⁿ⁻¹

Derivative Rule [Chain Rule]:
`\displaystyle (d)/(dx)[f(g(x))] =f'(g(x)) \cdot g'(x)`

Explanation:

Step 1: Define

Identify

`\displaystyle H(x) = \sqrt[3]{F(x)}`

Step 2: Differentiate

1. Rewrite function [Exponential Rule - Root Rewrite]:
   `\displaystyle H(x) = [F(x)]^\bigg{(1)/(3)}`
2. Chain Rule:
   `\displaystyle H'(x) = (d)/(dx) \bigg[ [F(x)]^\bigg{(1)/(3)} \bigg] \cdot (d)/(dx)[F(x)]`
3. Basic Power Rule:
   `\displaystyle H'(x) = (1)/(3)[F(x)]^\bigg{(1)/(3) - 1} \cdot F'(x)`
4. Simplify:
   `\displaystyle H'(x) = (F'(x))/(3)[F(x)]^\bigg{(-2)/(3)}`
5. Rewrite [Exponential Rule - Rewrite]:
   `\displaystyle H'(x) = \frac{F'(x)}{3[F(x)]^\bigg{(2)/(3)}}`

Step 3: Evaluate

1. Substitute in x [Derivative]:
   `\displaystyle H'(5) = \frac{F'(5)}{3[F(5)]^\bigg{(2)/(3)}}`
2. Substitute in function values:
   `\displaystyle H'(5) = \frac{6}{3(8)^\bigg{(2)/(3)}}`
3. Exponents:
   `\displaystyle H'(5) = (6)/(3(4))`
4. Multiply:
   `\displaystyle H'(5) = (6)/(12)`
5. Simplify:
   `\displaystyle H'(5) = (1)/(2)`

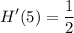
Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Derivatives

Book: College Calculus 10e