Question

Write the equation of the line fully simplified slope-intercept form.

Answer 1

The equation of the line in slope-intercept form is y = -x + 6

Explanation:

The form of the slope-intercept form of the linear equation is

y = m x + b, where

• m is the slope of the line

• b is the y-intercept

The rule of the slope is
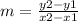`m=(y2-y1)/(x2-x1)` , where

• (x1, y1) and (x2, y2) are two points on the line

Let us choose two points on the line to form the equation

∵ Points (6, 0) and (0, 6) lie on the line

∴ x1 = 6 and y1 = 0

∴ x2 = 0 and y2 = 6

→ Substitute them in the rule of the slope to find it

∵
`m=(6-0)/(0-6)=(6)/(-6)=-1`

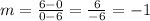
∴ m = -1

→ Substitute it in the form of the equation above

∵ y = -1(x) + b

∴ y = -x + b

∵ b is the y-intercept

∵ The y-intercept is the value of y at x = 0

∵ At x = 0, y = 6

∴ b = 6

→ Substitute the value of b in the equation

∵ y = -x + 6

∴ The equation of the line in slope-intercept form is y = -x + 6