GiveN:
- The coordinate of the point P(4 , 3)
- Coordinate of origin O(0,0)
What to find?
- Distance between P and O i.e. origin
Step-wise-Step Explanation:
We can find the distance between the coordinate of P and the origin O by using distance formula.
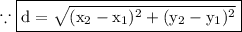
Plugging the given values of x1, x2 and y1, y2:
⇒
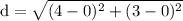
This can be written as,
⇒
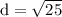
And the distance will be:
⇒
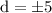
But distance is a scalar quantity, and can't be negative. Hence the distance between the point P and the origin is 5 units.