Answer:
0.309
Explanation:
→ Utilise the binomial distribution formula
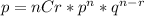
→ It first will be 5C2 as there is 5 possible voters but he wants 2 voters
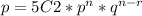
→ Then it will be
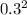 as the probability they are in favour is 0.3 and we get the n from the 2 number for the 5C2
as the probability they are in favour is 0.3 and we get the n from the 2 number for the 5C2
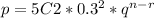
→ There for q will be 0.7 as it is the other probability left and the power will be 3 as it is n - r
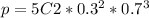
→ Then getting 0.3087 which is 0.309