Answer:
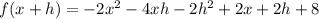

Explanation:
Given the function:
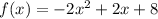
Find
- f ( x + h )
- f ( x + h ) - f ( x )
Recall the identity to square a binomial:
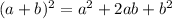
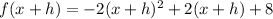
Squaring the binomial:
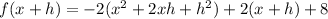
Multiplying:
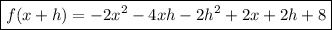
--------------------------------------------------------------
To compute f ( x + h ) - f ( x ), we use the last result:
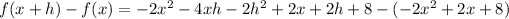
Multiplying:
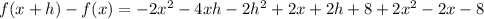
Simplifying similar terms:
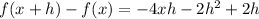
Factoring:
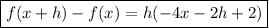
Note: This expression is commonly used to compute the first derivative of a function by its definition.