Question

Which statements accurately describe how to determine the y-intercept and the slope from the graph below? On a coordinate plane, a line goes through points (0, negative 6) and (3, 0). To find the y-intercept, begin at the origin and move horizontally to the graphed line. To find the slope, use two ordered pairs on the line and substitute into the equation m = StartFraction y 2 minus y 1 Over x 2 minus x 1 EndFraction. To find the y-intercept, begin at the origin and move horizontally to the graphed line. To find the slope, use two ordered pairs on the line and substitute into the equation m = StartFraction x 2 minus x 1 Over y 2 minus y 1 EndFraction.

Answer 1

answer is C.

Explanation:

Answer 2

Answer: To find the y-intercept, begin at the origin and move vertically to the graphed line.

To find the slope, use two ordered pairs on the line and substitute into the equation
`m=\frac{{y_{2-y_(1) } } }{x_{2-x_(1) } }`

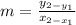
To find the x-intercept, begin at the origin and move horizontally to the graphed line.

Explanation:

There seems to be a typographical error in the answer choices.

To find the y-intercept you move vertically, up or down the y-axis.

To find the x-intercept you move horizontally, right or left the x-axis.

I made the corrections in my answer.

The description of the equation in the answers, "m = StartFraction y 2 minus y 1 Over x 2 minus x 1 EndFraction." looks like

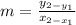`m=\frac{{y_{2-y_(1) } } }{x_{2-x_(1) } }` It means to put the difference in y-values 0 -(-6) in the numerator

and put the difference in the x-values in the denominator 3 - 0. Simplify, Then divide.

I hope this helps!