Question

Find the equation of the perpendicular bisector of AB when A(-7,2) and B(3,4)

Answer 1

Given:

Two points are A(-7,2) and B(3,4).

To find:

The perpendicular bisector of AB.

Solution:

Slope formula:

`m=(y_2-y_1)/(x_2-x_1)`

Slope of AB is

`m_1=(4-2)/(3-(-7))`

`m_1=(2)/(3+7)`

`m_1=(2)/(10)`

`m_1=(1)/(5)`

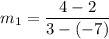
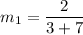
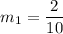
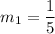
Procut of slopes of perpendicular line is -1.

So, slope of perpendicular bisect is opposite of reciprocal of
`(1)/(5)`.

`m_2=-5`

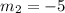
Midpoint of AB is

`Midpoint=\left((x_1+x_2)/(2),(y_1+y_2)/(2)\right)`

`Midpoint=\left((-7+3)/(2),(2+4)/(2)\right)`

`Midpoint=\left((-4)/(2),(6)/(2)\right)`

`Midpoint=\left(-2,3\right)`

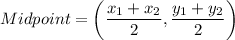
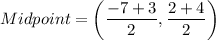
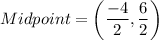
Slope of perpendicular bisector is -5 and it passes through (-2,3), so the equation of perpendicular bisector is

`y-y_1=m(x-x_1)`

where, m is slope.

`y-3=-5(x-(-2))`

`y-3=-5(x+2)`

`y-3=-5x-10`

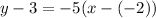
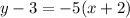
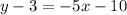
Add 3 on both sides.

`y=-5x-10+3`

`y=-5x-7`

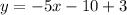
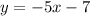
Therefore, the equation of perpendicular bisector is
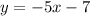`y=-5x-7`.