Question

(a)A three-point transverse bending test is conducted on a cylindrical specimen of aluminum oxide having a reported flexural strength of 300MPa (43,500psi). If the specimen radius is 5.0mm (0.20in.) and the support point separation distance is 15.0mm (0.61in.), would you expect the specimen to fracture when a load of 7500N (1690lbf) is applied? Justify your answer.

Answer 1

The specimen would not fracture having a flexural strength ( 300 MPa ) > ( 286.5 MPa )

Step-by-step explanation:

The expression to represent the stress on a cylindrical specimen by a three-point transverse bending test

б =
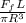`(F_(f)L )/(\pi R^3)` ---------------- ( 1 )

where : R = radius, Ff = flexural load,

L = distance between loads ( two loads )

Given data:

7500 N = flexural load

(15 * 10^-3) m = distance between loads

(5 * 10^-3) m = radius

input the given values into equation 1

б = 286.5 * 10^6 N/m^2 = 286.5 MPa

Note : the flexural strength of the cylindrical specimen is 300MPa

Therefore the specimen would not fracture having a flexural strength ( 300 MPa ) > ( 286.5 MPa )