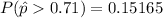Complete Question
The complete question is shown on the first uploaded image
Answer:
a

b
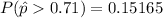
Explanation:
From the question we are told that
The population proportion is

The sample size is n = 50
Generally the mean of this sampling distribution is
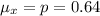
Generally the standard deviation is mathematically represented as
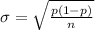
=>
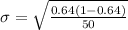
=>

Generally the probability that the value of
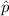 will be between 0.54 and 0.61 is mathematically represented as
will be between 0.54 and 0.61 is mathematically represented as
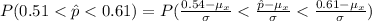

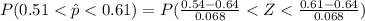
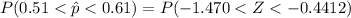
=>
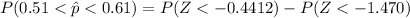
From the z table the probabilities of ( Z < -0.4412 ) and (Z < -1.912 ) is

and
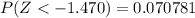
Generally
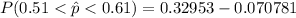

Generally the probability that the value of
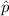 will be greater than 0.71 is mathematically represented as
will be greater than 0.71 is mathematically represented as
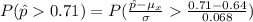
=>
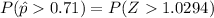
From the z table the probabilities of ( Z > 1.0294 )
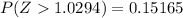
So