Answer:
The amount invested on 5.6% interest = $80488
The amount invested on 9.7% interest = $69512
Explanation:
Given that total amount of investment = $150,000
Let the amount invested on 5.6% interest = $

Now, amount invested on 9.7% interest = $(150000-
 )
)
Total interest to be earned = 7.5%
Formula to be used:
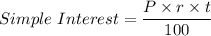
Where,
 is the amount invested
is the amount invested
 is the rate of interest
is the rate of interest
 is the time for which amount is invested.
is the time for which amount is invested.
As per question statement:
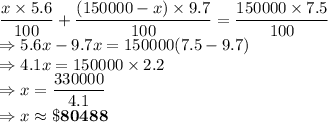
The amount invested on 5.6% interest = $80488
The amount invested on 9.7% interest = $150000 - $80488 = $69512