Question

Two containers are leaking water.Container A Leaked at a rate of 2/3 a gallon every 1/4 an hour. Container B leaked at a rate of 3/4 a gallon every 1/3 an hour. Which container is is losing water faster?

Answer 1

container A is losing water faster

Explanation:

Given;

the rate of water loss in container A = 2/3 a gallon every 1/4 an hour

the rate of water loss in container B = 3/4 a gallon every 1/3 an hour

Determine the rate of water loss for each container;

Container A:

Volume of water loss, V = 2/3 a gallon

time of water loss, t = 1/4 an hour = 15 minutes

rate of water loss, Q = V/t

`Q = ((2 )/(3)\ gallon) *((1)/(15 \ mins) )\\\\Q = (2)/(45)((gallon)/(mins)) = 0.0444 \ gpm`

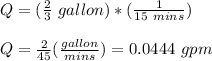
Container B:

Volume of water loss, V = 3/4 a gallon

time of water loss, t = 1/3 an hour = 20 minutes

rate of water loss, Q = V/t

`Q = ((3)/(4) \ gallon)*((1)/(20 \ mins))\\\\Q = (3)/(80) ((gallon)/(mins)) = 0.0375 \ gpm`

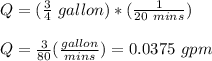
The rate of container A is higher than container B, thus container A is losing water faster