Question

S.schoology.com/assignment/3264134626/assessment

Question 15 (1 point)
How many sides does a regular polygon have if each interior angle measures 1757
a
72
38
ОООО
0 0 0 0
70
5

Answer 1

The regular polygon has 72 sides ⇒ A

Explanation:

The rule of the measure of an interior angle of a regular polygon is:

The measure of each angle =
`((n-2)180)/(n)` , where n is the number of its sides

Let us use this rule to solve the question

∵ The polygon is regular

∴ All sides are equal in lengths

∴ All interior angles are equal in measures

∵ The measure of an interior angle of the regular polygon is 175°

→ Substitute it in the rule above to find n

∴ 175° =
`((n-2)180)/(n)`

→ Multiply both sides by n to cancel the denominator in the right side

∴ 175 n = (n - 2)180

→ Multiply the bracket by 180

∴ 175 n = n(180) - 2(180)

∴ 175 n = 180 n - 360

→ Subtract 180 n from both sides

∵ 175 n - 180 n = 180 n - 180 n - 360

∴ - 5 n = - 360

→ Divide both sides by -5

∵
`(-5n)/(-5)=(-360)/(-5)`

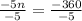
∴ n = 72

∴ The regular polygon has 72 sides