Answer:
The margin of error reduces to half of it original size
Explanation:
From the question we are told the confidence level is 95% , hence the level of significance is

=>

Generally from the normal distribution table the critical value of
 is
is
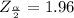
Generally the margin of error is mathematically represented as
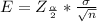
Let assume the standard deviation is
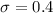
When sample size is n = 25


When sample size is n = 100
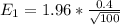
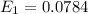
So
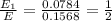
Hence the margin of error reduces to half of it original size