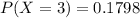Question

Customers arrive to a bank according to a Poisson process having a rate of 8.6 customers per hour. Suppose we begin observing the bank at some point in time. (a) What is the probability that 3 customers arrive in the first 30 minutes

Answer 1

The value is
`P(X = 3) = 0.1798`

Explanation:

From the question we are told that

The rate is
`\lambda = 8.6 \ hr ^(-1)`

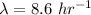
General probability distribution for Poisson distribution is mathematically represented

`P(X = x) = ((\lambda * t)^(x) )/(x!) * e^(-\lambda * t)`

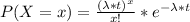
Given that is in per hour then the unit of t is in hours

Generally 30 minutes =
`0.5 \ hours`

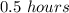
Generally the probability that 3 customers arrive in the first 30 minutes is mathematically represented

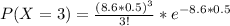
`P(X = 3) = (( 8.6 * 0.5 )^(3) )/(3!) * e^(-8.6 * 0.5 )`

=>
`P(X = 3) = 0.1798`