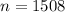Question

in order to prepare a production plan for the next buisness quarter, a purchasing agent needs to estimate the percentage of businesses that plan to buy office furnature in the next 90 days. How many randomly selected companies does she have to srvey to create an estimate in which we are 98% confident with a margin of 3%

Answer 1

The value is
`n =1508`

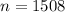
Explanation:

From the question we are told that

The margin of error is
`E = 0.03`

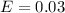
Here we will assume that sample proportion of businesses that plan to buy office furniture in the next 90 days to be
`\^ p = 0.5`

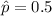
From the question we are told the confidence level is 98% , hence the level of significance is

`\alpha = (100 - 98 ) \%`

=>
`\alpha = 0.02`

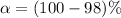
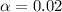
Generally from the normal distribution table the critical value of
`(\alpha )/(2)` is

`Z_{(\alpha )/(2) } =  2.33`

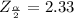
Generally the sample size is mathematically evaluated as

`n =[ \frac{ Z_{(\alpha )/(2) } }{E}] ^2 * [\^ p (1 - \^ p )]`

=>
`n =[ ( 2.33)/(0.03)] ^2 * [0.5 (1 - 0.5 )]`

=>
`n =1508`