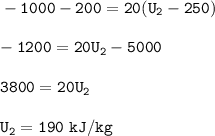Question

A closed system of mass 20 kg undergoes a process in which there is a heat transfer of 1000 Q6: ki from the system to the surroundings. The work done on the system is 200 kl. If the initial 5 specific internal energy of the system is (250+R:) kl/kg, what is the final specific internal energy, in kj/kg? Neglect changes in kinetic and potential energy:

Answer 1

The final specific internal energy : 190 kJ/kg

Further explanation

The laws of thermodynamics 1 state that: energy can be changed but cannot be destroyed or created

The equation is:

`\tt E_(in)-E_(out)=\Delta E~system\\\\\Delta E=\Delta U+\Delta KE+\Delta PE\\\\\Delta U=m(U_2-U_1)\\\\Q-W=\Delta U+\Delta KE+\Delta PE`

Energy owned by the system is expressed as internal energy (U)

This internal energy can change if it absorbs heat Q (U> 0), or releases heat (U <0). Or the internal energy can change if the system does work or accepts work (W)

The sign rules for heat and work are set as follows:

• The system receives heat, Q +

• The system releases heat, Q -

• The system does work, W -

• the system accepts work, W +

A closed system of mass 20 kg⇒m=20 kg

Heat transfer of 1000 kJ from the system to the surroundings⇒Q=-1000 kJ

The work done on the system is 200 kJ⇒W=+200 kJ

The initial specific internal energy of the system is 250 kJ /kg⇒U₁ = 250 kj/kg

Neglect changes in kinetic and potential energy⇒ΔKE+ΔPE=0, so

Q-W = ΔU

Input in equation

`\tt -1000-200=20(U_2-250)\\\\-1200=20U_2-5000\\\\3800=20U_2\\\\U_2=190~kJ/kg`