Given that the radius of the puddle expands, originally, at a rate of 1/4 unit/second instead of 1/2 unit/second.
The current rate of expension of the radius,
\frac {r(t)}{t}=frac 1 4 unit/second ...(i)
The resultant composite function is area, A(r(t)).
The area of a circular region having radius r is,
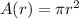
If the radius is the function of time, then the composite function is
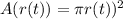
To determine the rate of change of area with respect to time, differentiate the composite function A(r(t)) once with respect to time, we have
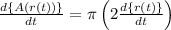
So, the composite function is changing twice of the rate of change of the radius.
By using equation (i), if r(i) is changing with R= 1/4 unit / second.
Then, A(r(t)) will change 2 x 1/4 = 1/2 square unit/second.