Step-by-step explanation:
A student measures the length of a magnesium ribbon three times and records the data as: (a). 23.486 cm, (b). 23.491 cm, (c). 23.511 cm
The labeled value of the magnesium ribbon is 23.490 cm
We need to find the precent error for all the data recorded by the student.
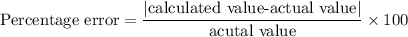
(a) When the calculated value = 23.486 cm
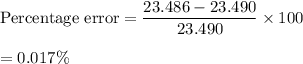
(b) When the calculated value = 23.491 cm
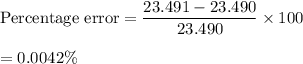
(c) When the calculated value = 23.511 cm
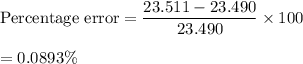
When the calculated value is 23.486 cm, it has least error of 0.017%.