Question

Laura was asked to estimate the volume of dirt in a large hill outside her school. She decides to model the hill using a truncated cone. She estimates that the hill has a base diameter of 80 feet, a top diameter of 40 feet, and a helght of 24 feet. What is the approximate volume of dirt in the hill?

Answer 1

70,336

Explanation:

Answer 2

The approximate volume of dirt in the hill = 70372 ft³

Explanation:

From the given information:

Laura uses a model of a truncated cone to estimate the volume of the dirt.

This implies that the volume of the dirt in the large hill took the shape of a truncated cone.

Her estimate then includes:

a base diameter = 80 feet ; then the base raidus (R) = 80 feet/2 = 40 feet

a top diameter = 40 feet ; then the top radius (r) = 40 feet/2 = 20 feet

a helght = 24 feet.

Using the formula for the volume of a truncated cone :

`V =(1)/(3) * \pi * h * ( r^2 +r * R+ R^2)`

`V =(1)/(3) * \pi * 24 * ( 20^2 +20 * 40+ 40^2)`

`V =25.133 * ( 400 +20 * 40+ 1600)`

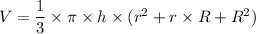
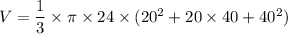
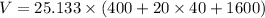
V = 70371.675 ft³

V ≅ 70372 ft³