Question

A furniture shop refinishes chairs. Employees use two methods to refinish chairs. Method I takes 1.5 hours and the material costs $9. Method II takes 2.5 hours, and the material costs $6. Next week, they plan to spend 198 hours in labor and $918 in material for refinishing chairs. How many chairs should they plan to refinish with each method?

Answer 1

Answer: They plan to refinish 82 chairs by method I and 30 chairs by method II.

Explanation:

Let x= Number of chairs refinished by method I.

y= Number of chairs refinished by method II.

As per given,
`1.5x+2.5y=198\ \ \ (i)`

`9x+6y=918\ \ \ \ (ii)`

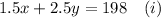
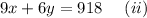
Multiply 6 on both sides of equation (i) , we get

`9x+15y=1188\ \ \ \ (iii)`

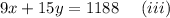
Subtract (ii) from (iii), we get

`9y=270\Rightarrow\ y=30`

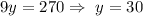
Put this in (ii),
`9x+6(110)=1188`

`\Rightarrow\ 9x+180=918\\\\\Rightarrow\ 9x=918-180\\\\\Rightarrow\ 9x=738\\\\\Rightarrow\ x=82`

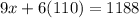
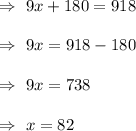
Hence, they plan to refinish 82 chairs by method I and 30 chairs by method II.