Answer:
Length =
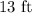
Width =
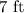
Explanation:
Let
Breadth of the garden = x
According to the question length of the garden = 6+x
Fencing given for the rectangular garden = 27 ft
Perimeter of the garden is given by

Here the garden's length will be along the garage so fencing will not done there. So length will be added only once in the perimiter.
The length of the garden is
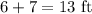 and the width is
and the width is
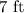 .
.