Question

g You believe that the mean life is actually less than the 100 hours the company claims. You decide to collect data on the average battery life (in hours) of a random sample and obtain the following information: the sample mean is 98.5, the standard error of the mean is 0.777 and the number of observations is 20. What can you conclude:

Answer 1

The mean life is actually less than the 100 hours the company claims.

Explanation:

In this case we need to test whether the mean life is actually less than the 100 hours the company claims.

The information provided is:

`n=20\\\bar x=98.5\\s=0.777`

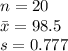
The hypothesis for the test can be defined as follows:

H₀: The mean life 100 hours, i.e. μ = 100.

Hₐ: The mean life is actually less than the 100 hours, i.e. μ < 100.

Assume the significance level as 5%.

As the population standard deviation is not known we will use a t-test for single mean.

Compute the test statistic value as follows:

`t=(\bar x-\mu)/(s/√(n))=(98.5-100)/(0.777)=-1.93`

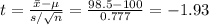
Compute the p-value of the test as follows:

`p-value=P(t_(n-1)<-1.93)=P(t_(19)<-1.93)=0.034`

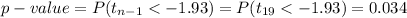
*Use a t-table.

Thus, the p-value of the test is 0.034.

Decision rule:

If the p-value of the test is less than the significance level then the null hypothesis will be rejected and vice-versa.

p-value = 0.034 < α = 0.05

The null hypothesis will be rejected at 5% level of significance.

Thus, concluding that the mean life is actually less than the 100 hours the company claims.