Complete Question
The complete question is shown on the first uploaded image
Answer:
The value is

Explanation:
From the question we are told that
The total number of students is n = 111
The total number of senior nutrition major k = 19
The total number of junior nutrition major is u = 19
Generally the probability that a senior Nutrition major and then a junior Nutrition major are chosen at random is mathematically represented as
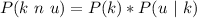
Here
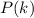 is the probability that a senior nutrition major is selected , this is mathematically represented as
is the probability that a senior nutrition major is selected , this is mathematically represented as
![P(k) = (k)/(n)[\tex]</p><p>=> [tex]P(k) = ( 19)/(111)[\tex] </p><p> and P( u | k) is the probability that a junior Nutrition major is selected given that a senior nutrition major has already been selected and this is mathematically represented as </p><p> [tex]P( u | k) = (u)/(n-1)]()
=>
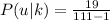
=>
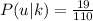
So
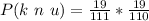
=>
