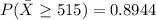Answer:
The value is
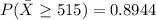
Explanation:
From the question we are told that
The mean is

The standard deviation is
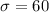
The sample size is n = 25
Generally the standard error of mean is mathematically represented as

=>

=>
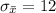
Generally the probability that a random sample of size 25 selected from the population will have a sample mean greater than or equal to 515 is mathematically represented as

Here
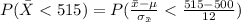
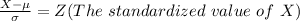

From the z table the probability of (Z < 1.25 )
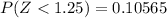
So
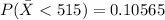
So
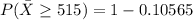
=>