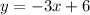Question

Write a linear equation in slope intercept from that is perpendicular to x−3y=3 and passes through the point (5, −9).

Answer 1

5

Explanation:

Answer 2

`y=-3x+6`

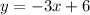
Explanation:

First, let’s determine the slope of the original equation.

We have
`x-3y=3`

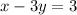
Subtract x from both sides and then divide both sides by -3. So:

`-3y=-x+3\\y=(1)/(3)x-1`

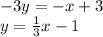
Therefore, the slope of our original line is 1/3.

Remember that perpendicular lines have slopes that are negative reciprocals of each other.

Therefore, the slope of our new perpendicular line is the negative reciprocal of 1/3.

Thus, the slope of our new line will be -3. We flip the fraction and add a negative.

So, the slope of our new line is -3. We also know that it passes through the point (5, -9).

Now, we can use the point-slope form:

`y-y_1=m(x-x_1)`

Where m is the slope and (x₁, y₁) is a point.

So, substitute -3 for m and (5, -9) for (x₁, y₁). This yields:

`y-(-9)=-3(x-5)`

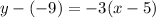
We want our line in slope-intercept form. So, distribute the right:

`y+9=-3x+15`

Subtract 9 from both sides. Therefore, our equation is:

`y=-3x+6`