Answer:
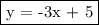
Explanation:
You are given the slope and the y-intercept of the line; so you can substitute these values into slope-intercept form:
 ;
;
- where
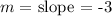
- and
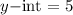
Plugging these values into slope-intercept form gives:
Another way to find the slope-intercept form of a line given the slope and a point:
We are given the slope and a point that the line passes through, so we can use the point-slope equation to find the slope-intercept form of the line.
The point that the line passes through is the y-intercept:
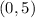 .
.
Point-slope form:
where
 are the coordinates of the point that the line passes through and
are the coordinates of the point that the line passes through and
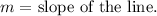
Substitute
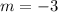 and
and
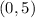 into the point-slope form equation.
into the point-slope form equation.
Simplify the equation on both sides.
Add 5 to both sides of the equation.
This is in slope-intercept form:
 , so we are done.
, so we are done.
The answer is
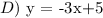 .
.