Question

The system of linear equations -2x+y=8 and -3x-y=7

Answer 1

Answer: x=-3, y=2

Step-by-step explanation: -2x+y=8 + -3x-y=7 and you get x=-3 then you put that in to x and pick one of the beginning equations i chose to put -3 in to -2(-3)+y=8 and got 6+y=8 then you subtract the 6 on both sides and should get y=2

Answer 2

(-3,2)

Explanation:

You can solve it by various methods. These two equations can be solved simultaneously or by matrix method, or even by graphing

Simultaneous is easier so lets get started.

1st equation :
`-2x+y=8`

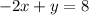
2nd equation:
`-3x-y=7`

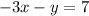
We solve it by using the substitution method which means there are two variables x and y and we substitute one variable in form of another variable.

Like, take the 1st equation

`-2x+y=8\\y=8+2x`

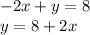
the equation is arranged in a different manner but its the same thing.

This new equation becomes our new third equation and now we simply put our third equation in our 2nd equation. Like this, take the 2nd equation and substitute it with our 3rd third equation

`-3x-y=7\\-3x-7=y\\-3x-7=8+2x\\-3x-2x=8+7\\-5x=15\\x=-3`

we get x=-3 and then we put the value of x and in our third equation to get the value of y or we can simply put it in any equation 1st , 2nd , 3rd it will give the same answer. I chose third.

`y=8+2x\\y=8+2(-3)\\y=8-6\\y=2`

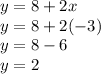
The solution set of our system of linear equations is

(-3,2)