(a)
![\bigcup\limits_(i=1)^7R_i=R_1=\boxed{[1,2]}](https://img.qammunity.org/2021/formulas/mathematics/college/th30ntg1fa417ghaj0a4l86ft8fu2r8q6l.png)
(b)
![\bigcap\limits_(i=1)^7R_i=R_7=\boxed{\left[1,\frac87\right]}](https://img.qammunity.org/2021/formulas/mathematics/college/y0w1697tt17eouucdrfi1ilz27wlrjgcug.png)
(c) No, because no two sets are disjoint. Why? Each of the
 contain the endpoint 1, so at the very least,
contain the endpoint 1, so at the very least,
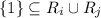 for
for
 .
.
(d)
![\bigcup\limits_(i=1)^nR_i=R_1=\boxed{[1,2]}](https://img.qammunity.org/2021/formulas/mathematics/college/1lza5n21jxoulqaejpnyzv26uzogwxzuqp.png)
(e)
![\bigcap\limits_(i=1)^nR_i=R_n=\boxed{\left[1,\frac{n+1}n\right]}](https://img.qammunity.org/2021/formulas/mathematics/college/enccjndcty8zdtf7auj8q0y1lopxk9umrk.png)
(f)
![\bigcup\limits_(i=1)^\infty R_i=R_1=\boxed{[1,2]}](https://img.qammunity.org/2021/formulas/mathematics/college/9sh36kkhu8gv0s19exhhwmjmz1v9qc3mkj.png)
because as i gets larger, the set
 gets smaller. The infinite union will be equivalent to the largest set in the family of sets.
gets smaller. The infinite union will be equivalent to the largest set in the family of sets.
(g)
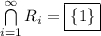
because 1 + 1/n converges to 1 as n goes to infinity, so
 converges to the singleton set {1} as i goes to infinity.
converges to the singleton set {1} as i goes to infinity.