Answer:
b)
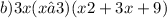
Step-by-step explanation:)
Take a factor
 from the expression. You're left with
from the expression. You're left with
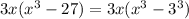 which is a difference of cubes. Cube differences are decomposed as a product as
which is a difference of cubes. Cube differences are decomposed as a product as
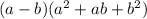 (an easy way to remember that is that you already have the minus in the first bracket to cancel out terms, so they're all pluses in the second).
(an easy way to remember that is that you already have the minus in the first bracket to cancel out terms, so they're all pluses in the second).