Question

A particular fruit's weights are normally distributed, with a mean of 230 grams and a standard deviation of 37 grams. If you pick 81 fruit at random, what is the probability that their mean weight will be between 231 grams and 240 grams

Answer 1

Answer: 0.3964

Explanation:

Given: A particular fruit's weights are normally distributed, with a mean
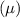`(\mu)` of 230 grams and a standard deviation
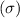`(\sigma)` of 37 grams.

Sample size : n= 87

Let
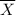`\overline{X}` be the sample mean.

The probability that their mean weight will be between 231 grams and 240 grams will be :

`P(231<\overline{X}<240)=P((231-230)/((37)/(√(81)))<\frac{\overline{X}-\mu}{(\sigma)/(√(n))},(231-230)/((37)/(√(81))))`

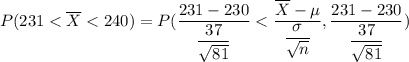
`=P(0.2432<Z<2.4324)\ \ \ \ [Z=\frac{\overline{X}-\mu}{(\sigma)/(√(n))}]\\\\=P(Z<2.4324)-P(Z<0.2432)\\\\=0.9925- 0.5961=0.3964`

Hence, the required probability = 0.3964