Answer:
density = 0.071984 g/cm³
Step-by-step explanation:
For a simple cubic lattice structure;
There are 8 atoms in the unit cell, with each edge of the cell contributing 1/8th atom to one unit cell.
Thus, in a unit cell, the required number of atoms = 8/8 = 1
The mass of the unit cell can be calculated by using the formula:

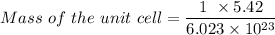
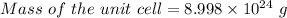
Similarly, given the atomic radius = 2.5 angstrom =
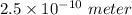
Thus, volume V = a³
The edge length for the simple cubic radius is:
a = 2 × r
a = 2 ×

a =
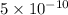 m
m
a =

Now;
volume V = a³
volume V =
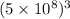
volume V =
 cm³
cm³
Finally; the density = mass/volume
density =
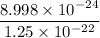
density = 0.071984 g/cm³