Question

Calculate the magnetic field at the center of a circular current loop of radius R divided by the magnetic field at a distance R away from a very long straight wire carrying the same current value I. (Note the loop and wire are not in electrical contact.)

Answer 1

The magnetic field at the center of a circular current loop of radius R divided by the magnetic field at a distance R away from a very long straight wire carrying the same current value I is
`\pi` or 3.14

Step-by-step explanation:

The magnetic field at center of a circular loop is given by

`B = (\mu _(o)I )/(2R)`

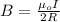
Where B is the magnetic field

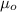`\mu _(o)` is the free space permeability constant (
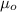`\mu _(o)` = 4π × 10⁻⁷ N/A²)

`I` is the current

and
`R` is the radius

For the magnetic field of a long straight wire, it is given by

`B = (\mu _(o)I )/(2\pi R)`

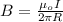
Where B is the magnetic field

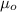`\mu _(o)` is the free space permeability constant (
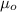`\mu _(o)` = 4π × 10⁻⁷ N/A²)

`I` is the current

and
`R` is the distance from the wire

Then, to calculate the magnetic field at the center of a circular current loop of radius R divided by the magnetic field at a distance R away from a very long straight wire carrying the same current value I, that will be

`(\mu _(o)I )/(2R) / (\mu _(o)I )/(2\pi R)`

=
`(\mu _(o)I )/(2R) * (2\pi R )/(\mu _(o)I)`

=
`\pi`

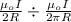
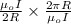
(NOTE:
`\pi` = 3.14)

Hence, the magnetic field at the center of a circular current loop of radius R divided by the magnetic field at a distance R away from a very long straight wire carrying the same current value I is
`\pi` or 3.14.