Question

The paraboloid z = 3 − x − x2 − 2y2 intersects the plane x = 1 in a parabola. Find parametric equations in terms of t for the tangent line to this parabola at the point (1, 3, −17). (Enter your answer as a comma-separated list of equations. Let x, y, and z be in terms of t.

Answer 1

the required parametric equation is:

x = 1

y = t + 3

z = -12t - 17

Explanation:

From the given information:

We are to express x,y, and z in terms of t.

The purpose of this question is to find the parametric equations in terms of t.

Given that the tangent line related to the parabola is the point (1,3, -17)

Provided that the paraboloid z = 3 - x - x² - 2y² intersect the plane x = 1 in a paraboloid; then:

z = 3 - 1 - (1)² - 2y²

z = 1 - 2y²

Suppose y = t, then the parametrization is given by:

(x (t), y(t), z(t) ) = (1, t, 1 - 2t²)

(x' (t), y'(t), z'(t) ) = (0,1, -4t)

At the point (x,y,z) = (1,3, -17)

For t (=y) = 3

Hence, (x'(3),y'(3),z'(3)) = (0,1, -12)

Therefore, the equation of the tangent line can be expressed as:

`(x-1)/(0)=(y-3)/(1)=(z-(-17))/(-12)=(t)`

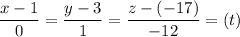
x - 1 = 0, y -3 = t, z + 17 = -12t

x = 1, y = t + 3 , z = -12t - 17

Thus, the required parametric equation is:

x = 1

y = t + 3

z = -12t - 17