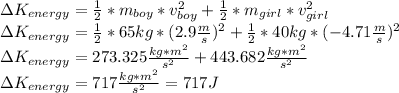Question

How much potential energy in the girl's body is converted into mechanical energy of the boy–girl system

Answer 1

The complete question is:

A 65 kg boy and his 40 kg sister, both wearing roller blades, face each other at rest. The girl pushes the boy hard, sending him backward with a velocity 2.90 m/s toward the west. Ignore friction."

How much potential energy in the girl's body is converted into mechanical energy of the boy–girl system?

Answer:

The ammount of potential energy converted to mechanical energy is 717 Joules

Step-by-step explanation:

Knowing the Law of Momentum Conservation we can state that the momentum change of the boy is equal to and opposite of the momentum change of the girl.

Written in a formula:

`m_(boy) *v_(boy) =-m_(girl) *v_(girl)`

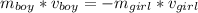
where

`m_(boy)\: \textup{is the boy's mass}\\v_(boy) \: \textup{is the boy's velocity}\\m_(girl) \: \textup{is the girl's mass}\\v_(girl) \: \textup{is the girl's velocity}`

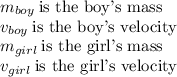
Solving for
`v_(girl)`:

`v_(girl)=(m_(boy) * v_(boy))/(-m_(girl))\\v_(girl)=(65kg * 2.9(m)/(s) )/(-40kg)\\v_(girl)=-4.71(m)/(s)`

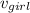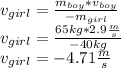
Finally, using the energy conservation formula:

`\Delta K_(energy) = (1)/(2)*m_(boy)* v_(boy)^(2) + (1)/(2)*m_(girl)* v_(girl)^(2)\\\Delta K_(energy) = (1)/(2)*65kg* (2.9(m)/(s))^(2) + (1)/(2)*40kg* (-4.71(m)/(s))^(2)\\\Delta K_(energy) = 273.325 (kg*m^(2))/(s^(2))+ 443.682(kg*m^(2))/(s^(2))\\\Delta K_(energy) = 717(kg*m^(2))/(s^(2)) = 717 J`