Question

A student uses the following steps to prove the sine sum identity. The proof is incorrect.

Answer 1

C. Step 4

Explanation:

got it right on edge :)

Answer 2

Step 4 is incorrect ⇒ C

Explanation:

Let us check step by step

∵ sin(x + y)

∵ sin(z) = cos(
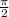`(\pi)/(2)` - z)

→ Replace z by (x + y)

∴ sin(x + y) = cos(
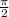`(\pi )/(2)` - [x + y])

∴ Step 2 is correct

∵ cos(
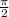`(\pi )/(2)` - [x + y]) = cos(
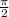`(\pi )/(2)` - x - y])

∵ take [
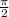`(\pi )/(2)` - x] as the 1st angle and y as the 2nd angle

∴ cos(
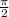`(\pi )/(2)` - x - y]) = cos([
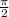`(\pi )/(2)` - x] - y)

∴ Step 3 is correct

∵ cos(a - b) = cos(a)cos(b) + sin(a)sin(b)

→ Replace a by ([
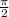`(\pi )/(2)` - x] and b by y

∴ cos([
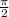`(\pi )/(2)` - x] - y) = cos(
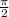`(\pi )/(2)` - x)cos(y) + sin(
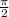`(\pi )/(2)` - x)sin(y)

∴ Step 4 is incorrect, the second angle is y not (-y)

∵ cos(
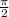`(\pi )/(2)` - x) = sin(x)

∵ sin(
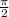`(\pi )/(2)` - x) = cos(x)

∴ cos(
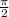`(\pi )/(2)` - x)cos(y) + sin(
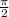`(\pi )/(2)` - x)sin(y) = sin(x)cos(y) + cos(x)sin(y)

∴ Step 5 is correct