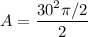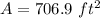Answer:
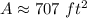
Explanation:
Area of a circular sector
A circular sector is defined by a central angle θ and the radius of the circle r. The area of the circular sector is:
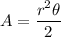
Note: θ must be expressed in radians.
The lawn sprinkler rotates θ=90° to an area of radius r=30 feet.
Expressing θ in radians:
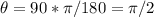
The area is: