Question

Write an equation of the line in​ point-slope form that passes through the given points.

​(4​,4​) and ​(5​,7​)
Please explain answer

Answer 1

Y - 4 = 3(X - 4)

Explanation:

Y2 - Y1 / X2 - X1 = M, 7 - 4 / 5 - 4 = M, 3 / 1 = M, M = 3

Y - Y1 = M(X - X1),

Y - 4 = 3(X - 4),

Y - 4 = 3X - 12,

+4 +4

Y = 3X - 8

Answer 2

Answer: y-4 = 3(x - 5)

Explanation:

The basic form for a point-slope equation is

y - b = m(x - a)

a is the x-value from the given coordinate, b is the y-value form the given coordinate, m is the slope. x and y are the variables in the function.

To find the slope, m, use the given coordinates. Get the difference in the y-values and divide by the difference in the x-values. This is "rise over run."

Given coordinate points: ​(4​,4​) and ​(5​,7​)
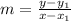`m=\frac{y-y_(1) } {x-x_(1 )}` substitute values:

m= 7-4/5-4 becomes m= 3/1 so the slope m = 3

To write the equation: Take the basic form, substitute b and a values from either given coordinate. And use the value of m we just calculated.

y - b = m(x - a) Using the second coordinate: (5,7)

y - 7 = 3(x - 5)

The attachment shows the graph of this equation.

(The equivalent slope-intercept form for this is y = 3x - 8 )