Question

The odds against a chess-master beating a computer are 11:5. What is the probability the at the chessmaster will win a match against the chessmaster?

Answer 1

Given:

The odds against a chess-master beating a computer are 11:5.

To find:

The probability the at the chessmaster will win a match against the computer?

Solution:

Let E be the event the chessmaster will win a match against the computer.

Odds against a chess-master beating a computer
`=(P(E'))/(P(E))`

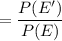
The odds against a chess-master beating a computer are 11:5. So,

`(P(E'))/(P(E))=(11)/(5)`

`P(E'):P(E)=11:5`

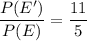
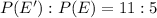
Let P(E') and P(E) are 11x and 5x respectively.

`P(E')+P(E)=1`

`11x+5x=1`

`16x=1`

`x=(1)/(16)`

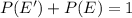
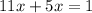
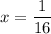
Now,

Probability the at the chessmaster will win a match against the computer P(E) is

`P(E)=5* (1)/(16)`

`P(E)=(5)/(16)`

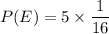
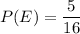
Therefore, the required probability is
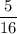`(5)/(16)`.