Question

Work out the length QR.

Give your answer correct to 3 significant figures.

Total marks: 5

Answer 1

The length of QR is 29.9 cm

Explanation:

Let us revise the sine and cosine rules

In triangle ABC:

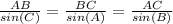`(AB)/(sin(C))=(BC)/(sin(A))=(AC)/(sin(B))` ⇒ sine rule

(AC)² = (AB)² + (BC)² - 2(AB)(BC)cos(∠B) ⇒ cosine rule

Let us use these rules to solve the question

In Δ PQS

∵
`(PS)/(sin(Q))=(QS)/(sin(P))`

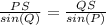
∵ PS = 11.7 cm

∵ m∠P = 95°, m∠Q = 28°

→ Substitute them in the rule above

∴
`(11.7)/(sin(28))=(QS)/(sin(95))`

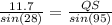
→ By using cross multiplication

∴ QS × sin(28) = 11.7 × sin(95)

→ Divide both sides by sin(28) to find QS

∴ QS = 24.82680292 cm

Now let us use the cosine rule to find QR

In Δ QSR

∵ (QR)² = (QS)² + (RS)² - 2(QS)(RS)cos(∠QSR)

∵ RS = 10.2 cm

∵ m∠QSR = 110°

→ Substitute these values in the rule above

∴ (QR)² = (24.82680292)² + (10.2)² - 2(24.82680292)(10.2)cos(110)

∴ (QR)² = 893.631984

→ Take √ for both sides

∴ QR = 29.89367799

→ Round it to 3 significant figures

∴ QR = 29.9 cm