Question

What frequency of rotation, in rpm, is required to give an acceleration of 1.4 g to an astronaut's feet, if her feet are 1.6 m from the platform's rotational axis

Answer 1

The frequency of rotation of the platform is approximately 27.970 revolutions per minute.

Step-by-step explanation:

In this case, the astronaut experiments a centrifugal acceleration as a reaction to the centripetal acceleration due to the rotation of the platform. (Second and Third Newton's Laws). Centripetal acceleration (
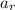`a_(r)`), measured in meters per second, is expressed as:

`a_(r) = \omega^(2)\cdot r` (Eq. 1)

Where:

`\omega` - Angular velocity, measured in radians per second.

`r` - Distance of the astronaut's feet from the rotational axis of the platform, measured in meters.

Now we clear the angular velocity:

`\omega = \sqrt{(a_(r))/(r) }`

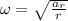
If we know that
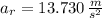`a_(r) = 13.730\,(m)/(s^(2))` and
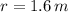`r = 1.6\,m`, the angular velocity of the platform is:

`\omega = \sqrt{(13.730\,(m)/(s^(2)) )/(1.6\,m) }`

`\omega \approx 2.929\,(rad)/(s)`

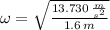
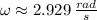
And this outcome is converted into revolutions per minute:

`\dot n = 2.929\,(rad)/(s)* (60\,s)/(1\,min)* (1\,rev)/(2\pi\,rad)`

`\dot n \approx 27.970\,(rev)/(min)`

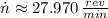
The frequency of rotation of the platform is approximately 27.970 revolutions per minute.