Question

Ali and Kiana buried a treasure together on their school's field. The actual field is 400 feet wide. Ali made an 8-inch-wide map to record its location. Kiana made her map using a scale of 1 in. To 20 ft. On Kiana's map, the treasure is 2 inches from the south edge of the field. How far is the treasure from the south edge on Ali's map?

Answer 1

The treasure is 0.8 inches from the south edge on Ali's map.

Explanation:

Scaling factor, f = (Original length)/(Scalet length)...(i)

Let
`f_1` and
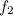`f_2` be the scaling factors used by Ali and Kiana respectively.

Given that the field is 400 feet= 400x12 inches wide and Ali made an 8-inch-wide map to record its location.

So,
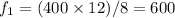`f_1 = (400*12)/8=600`...(ii)

Kiana made her map using a scale of 1-inch to 20 feet=20x12 inches.

So,
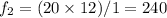`f_2=(20*12)/1=240`...(iii)

As on Kiana's map, the treasure is 2 inches from the south edge of the field,

so, from equations (i), and (ii), the original length of the treasure for the south edge of the field

`=2* f_2`

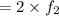
=2x240

=480 inches

Now, again from the equation (i) and (ii), the scaled length of the treasure on Ali's map

= 480/f_1

=480/600

=0.8 inches

Hence, the treasure is 0.8 inches from the south edge on Ali's map.