Question

Please answer this question​

Answer 1

`\bold{\huge{\underline{ Solution }}}`

Given :-

•
`\sf{ Polynomial :- ax^(2) + bx + c }`

• The zeroes of the given polynomial are α and β .

Let's Begin :-

Here, we have polynomial

`\sf{ = ax^(2) + bx + c }`

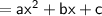
We know that,

Sum of the zeroes of the quadratic polynomial

`\sf{ {\alpha} + {\beta} = {(-b)/(a)}}`

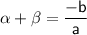
And

Product of zeroes

`\sf{ {\alpha}{\beta} = {(c)/(a)}}`

Now, we have to find the polynomials having zeroes :-

`\sf{ {\frac{{\alpha} + 1 }{{\beta}}} ,{\frac{{\beta} + 1 }{{\alpha}}}}`

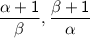
Therefore ,

Sum of the zeroes

`\sf{ ( {\alpha} + {\frac{1 }{{\beta}}} )+( {\beta}+{\frac{1 }{{\alpha}}})}`

`\sf{ ( {\alpha} + {\beta}) + ( {\frac{1}{{\beta}}} +{\frac{1 }{{\alpha}}})}`

`\sf{( {( -b)/(a)} ) + {\frac{{\alpha}+{\beta}}{{\alpha}{\beta}}}}`

`\sf{( {( -b)/(a)} ) + {(-b/a)/(c/a)}}`

`\sf{ {( -b)/(a)} + {(-b)/(c)}}`

`\bold{{( -bc - ab)/(ac)}}`

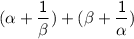
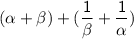
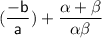
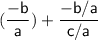
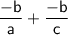
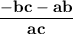
Thus, The sum of the zeroes of the quadratic polynomial are -bc - ab/ac

Now,

Product of zeroes

`\sf{ ( {\alpha} + {\frac{1 }{{\beta}}} ){*}( {\beta}+{\frac{1 }{{\alpha}}})}`

`\sf{ {\alpha}{\beta} + 1 + 1 + {\frac{1}{{\alpha}{\beta}}}}`

`\sf{ {\alpha}{\beta} + 2 + {\frac{1}{{\alpha}{\beta}}}}`

`\bold{ {(c)/(a)} + 2 + {( a)/(c)}}`

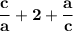
Hence, The product of the zeroes are c/a + a/c + 2 .

We know that,

For any quadratic equation

`\sf{ x^(2) + ( sum\: of \:zeroes )x + product\:of\: zeroes }`

`\bold{ x^(2) + ( {( -bc - ab)/(ac)} )x + {(c)/(a)} + 2 + {( a)/(c)}}`

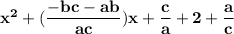
Hence, The polynomial is x² + (-bc-ab/c)x + c/a + a/c + 2 .

Some basic information :-

• Polynomial is algebraic expression which contains coffiecients are variables.

• There are different types of polynomial like linear polynomial , quadratic polynomial , cubic polynomial etc.

• Quadratic polynomials are those polynomials which having highest power of degree as 2 .

• The general form of quadratic equation is ax² + bx + c.

• The quadratic equation can be solved by factorization method, quadratic formula or completing square method.