Answer:
The net work per unit mass
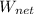 = 692.14 kJ/kg
= 692.14 kJ/kg
The thermal efficiency for the cycle =

Step-by-step explanation:
For an Ideal Rankine cycle:
The following properties were obtained from the steam tables at 1 MPa.
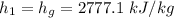
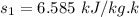
The properties obtained at 10 kPa are:
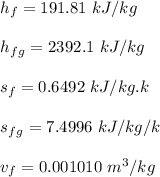
For 1 - 2 isentropic expansion:
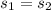
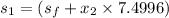
6.585 = 0.6492 + x₂ × 7.4996
6.585 - 0.6492 = 7.4996x₂
5.9358 = 7.4996x₂
x₂ = 5.9358/7.4996
x₂ = 0.791
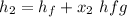
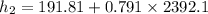
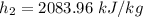
At 10 kPa;
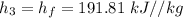
The pump work for the process:


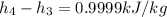
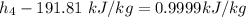
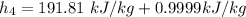
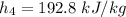
However, the turbine work
 can be computed by using the formula:
can be computed by using the formula:
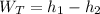
 ( 2777.1 - 2083.96 ) kJ/kg
( 2777.1 - 2083.96 ) kJ/kg
 693.14 \ kJ/kg
693.14 \ kJ/kg
Thus, the net work
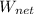 can be determined as:
can be determined as:
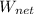 =
=

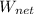 = 693.14 - 0.9999
= 693.14 - 0.9999
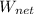 = 692.14 kJ/kg
= 692.14 kJ/kg
Similarly, to determine the thermal efficiency of this cycle, we need to first know the heat addition
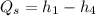

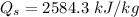
Finally, the thermal efficiency can be calculated by using the formula:
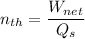
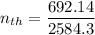
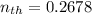
The thermal efficiency for the cycle =
