Question

Find the value of z so that the area under the standard normal curve from 0 to z is 0.4049 and z is positive.

Answer 1

1.31

Explanation:

The z score shows the number of standard deviations by which the raw score is above or below the mean. If the raw score is above the mean then the z score is positive but if the raw score is less than the mean then the raw score is negative. The z score is given by:

`z=(x-\mu)/(\sigma)\\ \\\mu=mean,\sigma=standard\ deviation,x=raw \ score`

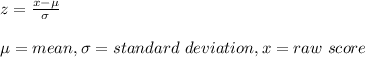
If the area under the standard normal curve from 0 to z is 0.4049, the total area = 0.4049 + 0.5 = 0.9049

From the normal distribution table, the z score that corresponds to a probability of 0.9049 = 1.31