Answer:
The size of the fund at the end of 7 years is $483.110
Step-by-step explanation:
Number of quarters = 4
We are given that the nominal rate of discount convertible quarterly is 4/41
Discount rate in each quarter =
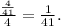
Let A is the value after discount and X is the original value:
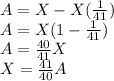
Now To calculate the value after 7 years we need to multiply each value by the interest raised to the correct power.
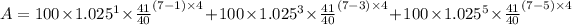
A=483.110
Hence the size of the fund at the end of 7 years is $483.110