Question

If she can throw each rock with a speed of 7.5 m/s relative to the ground, causing the wagon to move, how many rocks must she throw per minute to maintain a constant average speed against a 3.9-N force of friction

Answer 1

Complete question:

A child sits in a wagon with a pile of 0.64-kg rocks. If she can throw each rock with a speed of 7.5 m/s relative to the ground, causing the wagon to move, how many rocks must she throw per minute to maintain a constant average speed against a 3.9-N force of friction

Answer:

The number of rocks per minutes thrown is 49 rocks/min

Step-by-step explanation:

Given;

mass of the rock, m = 0.64 kg

speed of the rock, v = 7.5 m/s

frictional force,
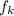`f_k` = 3.9 N

For an object to move at a constant speed, the applied force must be equal to the frictional force.

`f_k = N(F_a)\\\\f_k = N((mv)/(t))\\\\f_k = (N)/(t) (mv)\\\\(N)/(t) = (f_k)/(mv)`

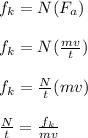
where;

`F_a` is the applied force

N/t is the number of rocks per minutes thrown

Substitute the given parameters;

`(N)/(t) = (f_k)/(mv)\\\\ (N)/(t) = (3.9)/(0.64*7.5)\\\\ (N)/(t) = 0.8125 \ (rocks)/(second) * (60 \ seconds)/(1 \ min) = 48.75 \ (rocks)/(min) \`

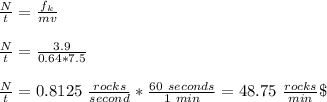
Approximately 49 rocks/min