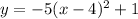Answer:

Explanation:
 - vertex form of equation of a parabola where vertex is (h,k)
- vertex form of equation of a parabola where vertex is (h,k)
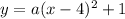 - vertex form of equation of a parabola where vertex is (4,1)
- vertex form of equation of a parabola where vertex is (4,1)
The parabola goes through point (3, -4) so if x=3 then y=-4

Therefore the equation of the parabola in vertex form with vertex (4,1), going through point (3,-4):